课程咨询

SAT考试网 美本资料下载 25考试辅导 0元讲座 考前测试

新东方在线 > SAT > SAT数学 > 正文
美国本科留学资料领取

扫码添加助教

免费领取

备考资料大礼包

扫码关注公众号

SAT数学知识讲解：三角形(2)

2013-08-09 16:33:52来源：互联网

　　下面新东方在线为大家整理了SAT数学知识讲解：三角形(2)，供考生们参考，以下是详细内容。

　　30-60-90 Triangles

　　The guy who named 30-60-90 triangles didn’t have much of an imagination. These triangles have angles of

　　, and

　　. What’s so special about that? This: The side lengths of 30-60-90 triangles always follow a specific pattern. Suppose the short leg, opposite the 30° angle, has length x. Then the hypotenuse has length 2x, and the long leg, opposite the 60° angle, has length x

　　. The sides of every 30-60-90 triangle will follow this ratio of 1:

　　: 2 .

SAT数学知识讲解：三角形(2)

　　This constant ratio means that if you know the length of just one side in the triangle, you’ll immediately be able to calculate the lengths of all the sides. If, for example, you know that the side opposite the 30o angle is 2 meters long, then by using the 1:

　　: 2 ratio, you can work out that the hypotenuse is 4 meters long, and the leg opposite the 60o angle is 2

　　meters.

　　And there’s another amazing thing about 30-60-90 triangles. Two of these triangles joined at the side opposite the 60o angle will form an equilateral triangle.

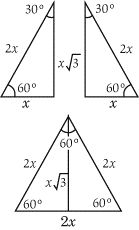
SAT数学知识讲解：三角形(2)

　　Here’s why you need to pay attention to this extra-special feature of 30-60-90 triangles. If you know the side length of an equilateral triangle, you can figure out the triangle’s height: Divide the side length by two and multiply it by

　　. Similarly, if you drop a “perpendicular bisector” (this is the term the SAT uses) from any vertex of an equilateral triangle to the base on the far side, you’ll have cut that triangle into two 30-60-90 triangles.

　　Knowing how equilateral and 30-60-90 triangles relate is incredibly helpful on triangle, polygon, and even solids questions on the SAT. Quite often, you’ll be able to break down these large shapes into a number of special triangles, and then you can use the side ratios to figure out whatever you need to know.

　　45-45-90 Triangles

　　A 45-45-90 triangle is a triangle with two angles of 45° and one right angle. It’s sometimes called an isosceles right triangle, since it’s both isosceles and right. Like the 30-60-90 triangle, the lengths of the sides of a 45-45-90 triangle also follow a specific pattern. If the legs are of length x (the legs will always be equal), then the hypotenuse has length x

SAT水平能力测试【0元免费测试】

本文关键字: SAT数学 SAT数学知识讲解

美本留学资料大礼包

微信扫描二维码 回复【美本资料】

机考SATCB官方样题｜可汗练习题｜AP全科大纲/备考资料包

更多资料

添加美本助教
即可获取美本资料大礼包

美本资料大礼包
  • 1.打开手机微信【扫一扫】，识别上方二维码；
  • 2.添加【美本助教】，回复上方关键字领取学习资料。
更多>>
更多内容
课程咨询 ACT课程 SAT课程 0元公开课 水平诊断

添加美本助教号

自动领取备考资料大礼包

1. 打开手机微信【扫一扫】，识别上方二维码；
2.添加【美本助教】，自动领取留学备考资料大礼包。

可汗学院新SAT题目完整版

微信扫描下方二维码 即可获取

SAT1500分录播课（旗舰版+1对1） 托福精讲录播课（旗舰版） 托福8-10人直播VIP小班
更多>>
更多公开课>>

2024美本留学资料免费领取

微信添加美本助教

新东方美本助教
更多>>
更多资料
我要
反馈 返回
首页
课程咨询 课程中心 0元讲座 SAT模考网站 托福TPO模考网站 SAT课程

添加新东方在线美本助教号

自动领取备考资料大礼包

1. 打开手机微信【扫一扫】，识别上方二维码；
2.添加【Ella助教】，自动领取留学备考资料大礼包。

  • 1
  • 2
  • 3
  • 4
  • 5
  • 6
  • 7
  • 8
  • 9
  • 10